
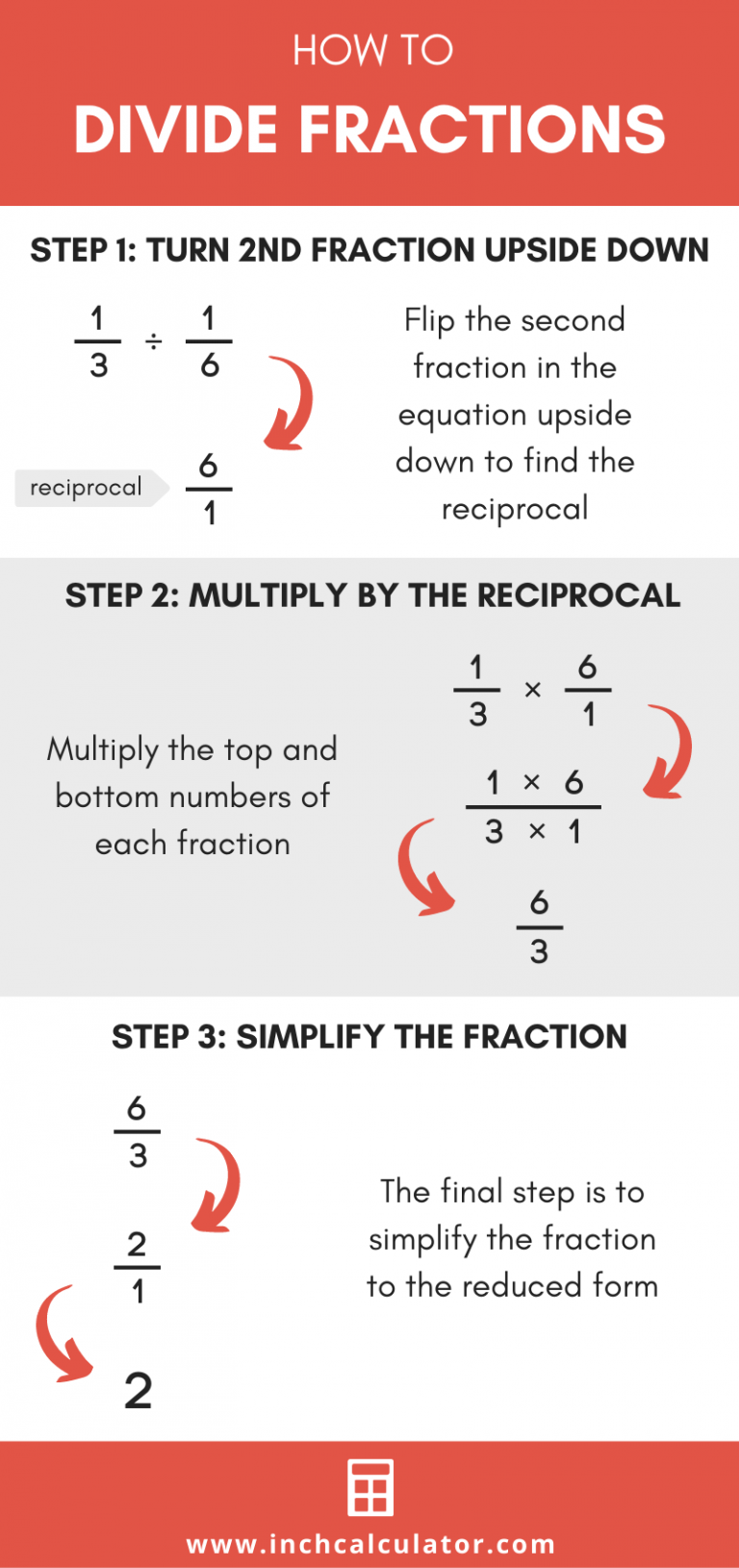
Fractions ≥ 7/8 are rounded up to 8/8=1Įstimating sums and differences of fractions to the nearest 1/8. Fractions ≥ 5/8 and < 7/8 are rounded to 6/8=3/4. Fractions ≥ 1/8 and < 3/8 are rounded to 2/8=1/4. See the section on " Value of Estimating Fractions" below.Įstimating sums and differences of fractions to the nearest 1/2Įstimating sums and differences of fractions to the nearest 1/4 For more precise estimating we added the ability to round fractions to the closest 1/4 or 1/8. This online calculator was originally set up to estimate by rounding fractions to the nearest 1/2. Use rounding to estimate answers when adding or subtracting proper fractions. Estimate sums and differences for positive proper fractions, n/d (numerator/denominator), where n ≤ d and 0 ≤ n/d ≤ 1. Flip the fraction we are dividing by (the divisor) so its numerator is on the bottom and its denominator is on the top. The general rule for dividing fractions is: From here we simply multiply the fractions together. ĭividing FractionsDividing fractions is very similar to multiplying fractions but includes an extra step, in which we swap the numerator and denominator of the divisor-the number by which we will divide the other fraction-to find its reciprocal. Multiply the denominators (bottom numbers). Multiply the numerators (top numbers). There are 4 steps to multiplying fractions: Multiplying fractionsThe general rule for multiplying fractions is: If the result was, for example, we would reduce it to. Simplify the resulting fraction by reducing, if possible, as described above in step 1. At this point, the fractions should have the same denominator, meaning we can simply add or subtract the numerators and write the result over the denominator we found in the previous steps. There are two ways to find the lcm: listing numbers' multiples (solver coming soon!) and by prime factorization. To do this, we find the least common multiple (lcm) of the denominators and use it as the common denominator. Multiply the top and bottom of each fraction by the denominator of the other fraction. There are two ways to find the common denominator:ġ. Find the fractions' common denominator. Another example is, which would reduce to. For example, is the largest number by which and can be evenly divided, so we can divide the numerator and denominator of by to reduce it to. The gcf of a set of numbers is the highest number that can divide evenly into all numbers in the set with no remainder. Divide the numerator (top number) and the denominator (bottom number) by their greatest common factor (gcf). Simplify the fractions by reducing them, if possible. .gif)
There are 4 steps to adding and subtracting fractions: The general rule for subtracting fractions is: Īdding and subtracting fractionsThe general rule for adding fractions is: We would then add this to the numerator,, to get, which we would place over the original denominator,, to get.

For example, in converting to an improper fraction, we would multiply the denominator,, by the whole number,, to get.
To convert a mixed fraction into an improper fraction, multiply the denominator (bottom number) by the whole number (number in front or to the left of the fraction), add the product to the numerator (top number), and write the sum over the original numerator.To convert an integer into an improper fraction, simply place the integer over.When doing operations with fractions, it is usually easier to first convert any integers and/or mixed fractions into improper fractions: It is important to note that improper fractions and mixed fractions can be used to express the same values. Mixed fractionsA whole number combined with a proper fraction.Improper fractionsThe numerator is larger than the denominator.Proper fractionsThe numerator is smaller than the denominator.To express the fraction as a single number, the quotient, we divide the numerator by the denominator. A fraction represents a smaller part of a whole and is usually written as a numerator, which represents the smaller part, written over a denominator, which represents the whole.


.gif)





 0 kommentar(er)
0 kommentar(er)
